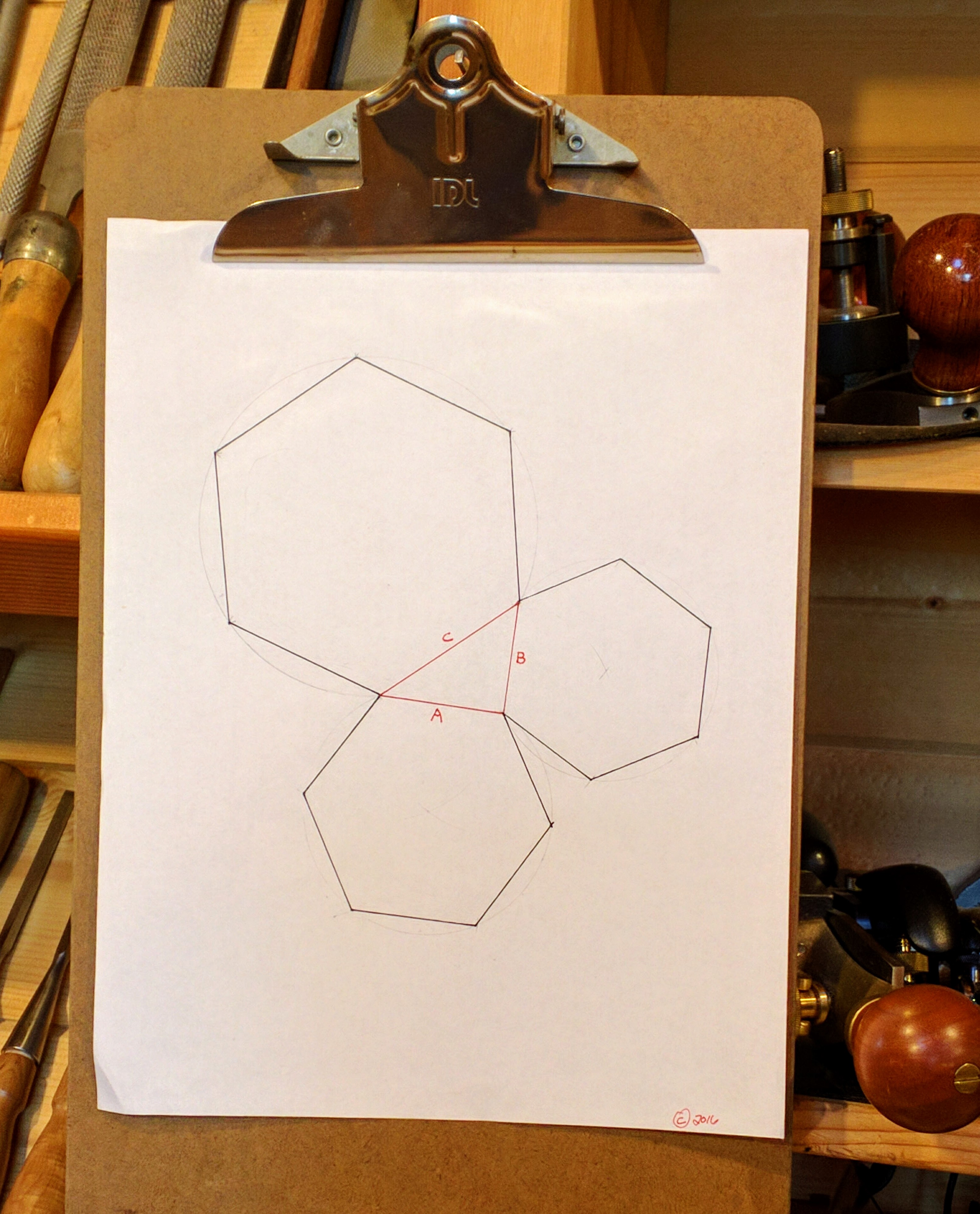
We (and our kids) have all been inoculated with enough Geometry during middle school to “know” the Pythagorean theorem. You know, the one that enables us to rattle off: “A squared plus B squared equals C squared.” But that particular manifestation of the underlying geometric truth of our particular universe isn’t limited to squares. In this drawing, we have three hexagons built upon the three legs (labeled A, B and C) of a right triangle. Just like squares, if you add the area of the two little shapes they will equal the area of the biggest one. In other words: A hexagoned plus B hexagoned equals C hexagoned. This works for all similar shaped polygons by the way.
Want the “proof”? All you need is a couple sticks and a bit of string as in the photo below. Have your four year old lend you a hand…she’ll immediately intuit what an equation is really all about! (No, this is not your rigorous algebraic proof, or even a Euclidean logic proof…Instead it’s what me bandmates used to call: “Good enough for rock and roll”.)